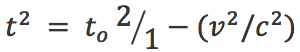Relativity
Motion through space is related to motion through time. Albert Einstein was the first person to recognize this relationship between space and time. In 1905, he published his special theory of relativity.
According to Einstein, space and time are components of space-time. When you stand still, you are traveling only through time but, when you are moving, you travel through space-time (space and time). If you travel at the speed of light, you travel only through space! Thus, no movement means maximum rate of travel through time and movement at the speed of light means time stands still. This slowing down of time with speed is called time dilation.
When you move through space, you affect the rate of moving into the future. This is the effect that a moving clock runs slower than an identical stationary one. Time dilation has been measured in a number of experiments. One experiment involved counting the number of muons (radioactive particles) at the top and bottom of mountains as they traveled to the earth at nearly the speed of light. More muons were counted at the bottom and the time to decay was longer which is consistent with relativity. Identical clocks have been used to compare time in motion versus rest. The moving clocks run slower than the stationary clocks. Physical processes are affected by the passing of time such as chemical and biological processes, however, the moving person cannot detect the change in time interval because all methods of measuring time are slowed by the same factor. All is relative. The equation for time dilation is:
Speed depends on the frame of reference or, the place where it is observed. An inertial frame is a frame of reference at constant velocity (non-accelerating). If you were in a car which was stationary and another car drove into your car, the impact would be much greater than if you were moving the same direction as the car which hit you. By the same token, the impact would be greatest if you were moving towards the car that hits you. Another example would be, if you were riding in an elevator and the cable snapped. All other objects in the elevator with you (same frame of reference) would appear to have no speed at all, however, to someone outside the elevator (different frame of reference), you would have considerable speed.
Every measurement of the speed of light from any frame of reference always comes out the same. The light from your headlights on your car would reach an observer at the same speed regardless of whether the observer is approaching or receding from your car. The fact that the speed of light is always constant unifies space and time.
Einstein advanced two postulates of special relativity:
1. All laws of nature are the same in all uniformly moving (inertial) frames of reference.
2. The speed of light in empty space will always have the same value regardless of the motion of the source or the observer.
Time travel has always been a subject of much debate. It would be necessary to time travel if we wanted to explore much of outer space. The nearest star besides our sun is 4 light-years away. A light year is the distance light travels in one year. The center of our Milky Way galaxy is 30,000 light-years away! Travel at speeds at or near the speed of light would mean that the astronauts may be gone 5 years while 1000 years elapses on Earth! At the speed of light, the journey to the center of our galaxy would take no time but 30,000 years would pass on earth! Time only moves forward hence, time travel is only possible into the future. We can see into the past but cannot travel backwards in time. Looking at the stars in the sky at night is seeing into the past. Some of the stars are so far away it has taken millions of years for the light to reach earth, thus we are seeing what was millions of years ago, not what is currently happening.
In addition to time, motion through space also affects momentum, length, and energy of moving objects. Space, as well as time, undergoes changes with motion. As the speed of an object approaches the speed of light, length approaches zero. Space contracts only in the direction of motion. The formula for length contraction is:
where L₀ is the length at rest; L is the length of the moving object as measure by the observer; v is the speed of the object as measured by the observer; and c is the speed of light.
Momentum can increase without any limit, even though speed cannot. The relativistic momentum of an object is:
where p is momentum; m is rest mass. Rest mass is constant no matter what speed the object moves at. This formula means that as velocity of an object approaches c, it would develop infinite momentum! Thus, anything with mass cannot reach the speed of light. From this, it appears that c is the speed limit for anything in the universe.
One remarkable conclusion from the special theory of relativity is that mass is simply a form of energy. Rest mass, in a sense, is a form of potential energy. The amount of rest energy is related to the mass by the equation:
A change in energy of any object is accompanied by a change it mass. Mass and energy are the same thing!
Kinetic energy for ordinary speeds is found from the equation KE = 1/2 mv². At speeds approaching the speed of light, kinetic energy is:
Review Questions:
1. Rafaela and Cassie are twins. Suppose Rafaela takes a ride in a spaceship to a planet 4 light years away, traveling at a constant speed of 0.80c. After arriving at the planet, Rafaela quickly turns around and immediately comes back at the same speed. (a) How much older is Cassie when Rafaela returns? (b) How much older is Rafaela when she returns? (c) Why isn't the reverse true?
a. 10 years
b. 6 years
c. Because Cassie remained in one realm of space-time while Rafaela experienced two.
2. Jimmy is 30 years old and has a daughter 6 years old. He leaves on a space bus and takes a 5 year (space bus time) round trip at 0.00c. How old will he and his daughter be when he returns?
Jimmy will be 35 years old while his daughter will be 41.4 years old.
3. Eric takes a ride in a spaceship moving at 0.8c to a planet 4 light years away. From his frame of reference, what distance in light-years does he travel to the distant planet?
2.4 light-years
4. From Earth, the distance to the center of our galaxy is 24,000 light-years. From the frame of reference of a photon of light traveling from Earth to the center of our galaxy, what is the distance?
zero
5. An electron, with a rest mass of 9.11 x 10-31 kg shoots down a 1 km long accelerator at an average speed of 0.95c. (a) From the electron's frame of reference, how long is the accelerator? (b) From the electron's frame of reference, how long does it take to make the trip?
a. 0.3 km
b. 10*-6 seconds
6. A 100 W lightbulb consumes 100 J of energy every second. How long could you burn that lightbulb from the energy in one penny? The mass of a penny is 0.003 kg. (Assume 100% conversion of mass to energy.)
2.7 x 10*12 s (88,000 years)
7. Dan and Dan are abducted by aliens. The spaceship travels at 90% the speed of light relative to the earth. Two SOS signals are sent from the spacecraft to the earth. The signals are sent 9 hours apart from their reference frame. What is the time interval between the two signals as measured by people on earth?
20.6 hours
8. Angel and Amanda go shopping in a spaceship for 50 light years at 0.9990c. How long does the trip take to (a) their parents here on earth, and (b) to either passenger?
a. 50 years
b. 2.24 years
9. For the situation in problem 8, what is the distance in light years that the spaceship measures to the destination as they are traveling at the speed of 0.999c?
2.24 light years
10. William is traveling in a train moving at rest with respect to the surface of the earth. (a) What does he measure as the time it takes for a quarter to drop 1.0 m to the florr of the railroad car? (b) What does an observer in another railroad car traveling at a constant 25 m/s measure for this time?
a. t = .45 s (use x = vt + (1/2)at*2 equation)
b. 1 - 3.5 x 10*-15
Honors:
11. What is the effective mass of an electron moving at 0.80c?
m = 15.2 x 10*-31 kg
12. Greg and Andy need to know the relative velocities and masses of their space ships. Each ship, therefore, has a 1.0 m bar painted on the side of the ship alongside their rest mass. As David passes by a ship, he measures this 1.0 m bar as 0.93 m. (a) What is David's relative velocity? (b) David also observes their rest mass printed as 365,000 kg. What is their mass relative to David? (c) What does Greg measure for David's 1.0 bar?
a. 0.37c
b. 392,000 kg
c. 0.93 m and 0.37c. David has the same relative speed to them as they have to him.